Multiplication Facts, are they really necessary?
- jessicathelearning
- Mar 21, 2022
- 4 min read
Updated: Mar 28, 2023
We always have a calculator, right!? The probability of us being spontaneously quizzed on multiplication facts is extremely low in adulthood. So many parents and students no longer see a need to memorize multiplication facts. I would argue that parents supporting students with homework or homeschooling parents preparing to teach their child multiplication need to understand how multiplication ties into math skills moving forward.

The hard truth is multiplication is a significant stepping stone in a student's math ability. As students move into the fourth and fifth grade, they are introduced to equations with multiple steps, and stopping on each step to draw out multiplication models or use a calculator makes each problem take forever. Students get lost in the process because these extra steps pull their attention away from the actual problem at hand.
You could compare it to reading a book that is too hard for you and having to stop every other word to use the dictionary. Your attention keeps being pulled in different directions, so your brain can't focus on the book you are trying to read. Frustration sets in, and eventually, you give up.
Teach your child multiplication in a way that helps develop their math skills.
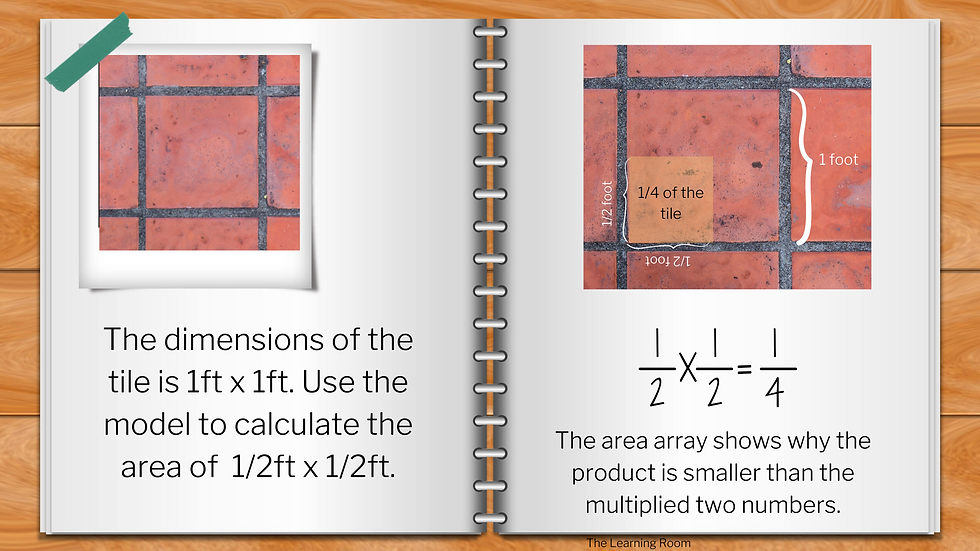
Don't get me wrong, memorizing multiplication facts is required you can download a list of our favorite tools to do that here. Still, teaching multiplication must be more than memorizing facts (we will talk about memorizing facts in a second). The common definition of multiplication is repeated addition, but more than repeated addition/skip-counting. We can't say students understand multiplication deeply if their understanding stops at repeated addition. As the math moves past the "repeated addition approach," students will not understand beyond the surface level. Consider ½ x ½, which is harder to explain with the repeated addition approach and instead is better explained by an area model. Understanding an array and area model is the ultimate goal as it leads to understanding multiplying fractions and calculating area.
Understanding Multiplication:
Repeated addition
Skip counting on a number line
Array
Area model
Typically, students need these concrete examples of multiplication longer than we think. The more students use models to solve multiplication problems, the more they understand multiplication beyond memorizing the facts. Multiplication models enhance students' ability to understand situations like why ½ x ½ = ¼, which is difficult for a young student to understand because, in whole number multiplication, the product (answer) is always larger than the numbers being multiplied together.
How can I support my student at home?
As your child develops their multiplication skills, encourage them to use models as long as necessary. They can use objects to make the models like above or draw them out on a sheet of paper. As their skills progress, you can encourage them to make area models on grid paper to create a visual model. This will add to the time it takes them to complete a problem, so it may be appropriate to decrease the number of problems they are working on if possible.
What does a deep understanding of multiplication look like?
Deep understanding sounds excellent! We all want our children to understand topics to master them and then apply them to the next skill, but what does that even mean? And how do we know if our child is at that level?
Students with a deep level of understanding of multiplication can do the following:
Multiplication fluency allows them to complete math problems without frustration.
They see the connection between multiplication and division.
Higher levels of understanding lead to:
Understanding an array leads to them understanding calculating area.
Learning to calculate rates is essential as rates lead to functions, slope, and everything in calculus.
Multiplication is wrapped into every skill students learn after third grade. Curriculums are written assuming that students have a strong understanding of multiplication and know their facts fluently. Learning multiplication may involve using flashcards but should also include other techniques.
I have created a foundation of understanding; now what?
Now that we understand multiplication and have some hands-on practice, it's time to memorize! This brings us back to our original question, why do we need to memorize the different facts? I gave you four ways to help multiply - no need to memorize anything - wrong.
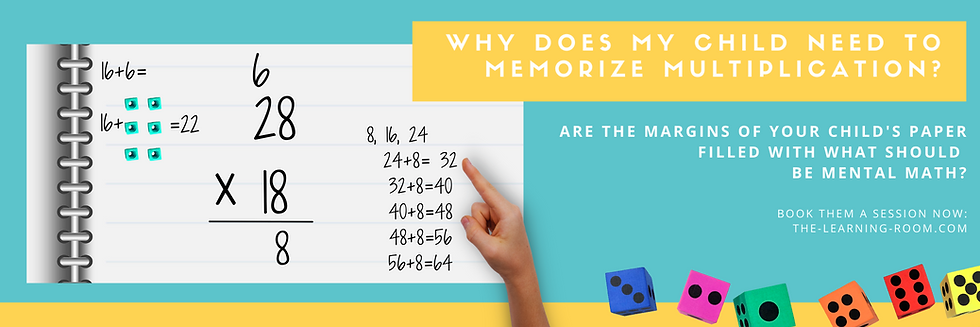
Imagine a student working on a word problem. Without multiplication fluency, they will spend a lot of time calculating simple multiplication in the margins eating up lots of time and energy. The more complicated the word problems get, the more mental math they will be doing in the margins and on their fingers. Eventually, the amount of simple math they are bogged down with prevents them from completing the problem.
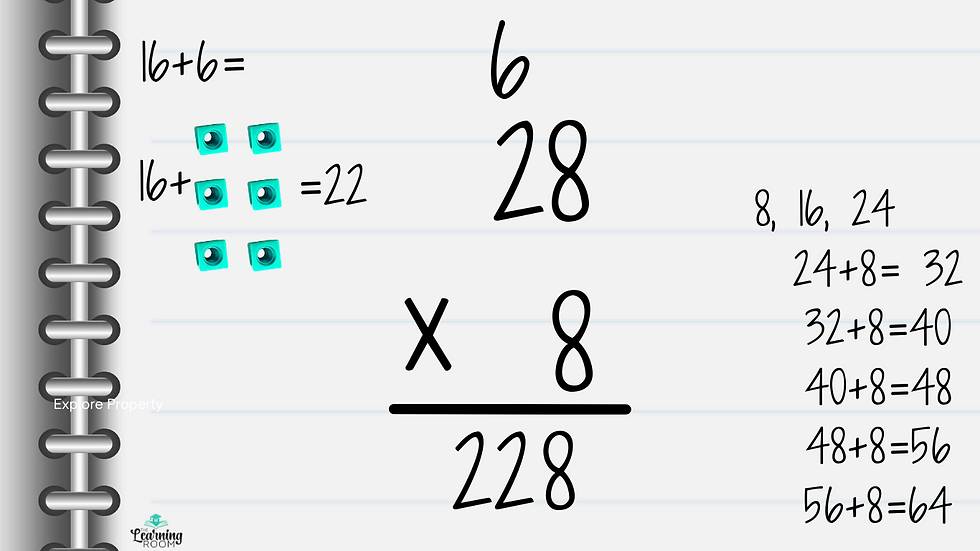
Having facts memorized allows students to work quickly and effectively. It also prevents students from "getting lost in the weeds," so to speak, because they are not constantly stopping to count on their fingers or calculate simple multiplication in the margins. These strategies will feel like they are working until the math problems become multi-step and have multiple parts. Parents typically call me for help around 4th or 5th grade saying the word problems overwhelm their students, and they are "so lost." I find that as soon as we have the multiplication under control, the word problems are not as overwhelming.
We created a list of all of our favorite resources to help students memorize their multiplication facts, download it here!
Do you feel like you have tried all the things? Is it still not working? Let us help. We offer a free assessment of your child's math ability and create a custom approach just for them, book a consultation today.
Image Credits:














Comments